Note: This is the first math game I ever invented. In the years since, I’ve become obsessed with math games. I started a weekly newsletter, Games for Young Minds, for parents of young kids who are looking for math games to play together. Check it out!
This past week I finally presented my integer lesson progression to math teachers in my district. It felt good to finally get a room full of people to grapple with the same topic I've been working on for most of this school year.
I'll be posting my lesson progression over the course of the next few weeks, but I decided to go ahead and share a little game that I use to practice fluency at the end of the unit. You can also use this with 8th graders and high schoolers who need integer practice but don't want to do yet another Kuta Software worksheet.
I call the game Integer Solitaire, but it can be played alone or in pairs. All you need is some poster board or big white boards and decks of cards.
The Rules
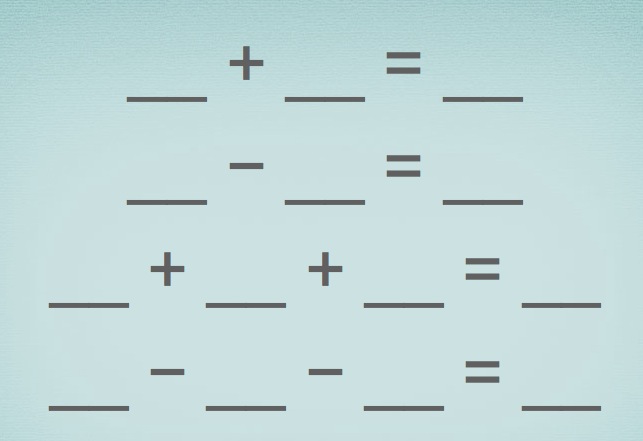
Give each kid a poster board or a big white board. The board needs to be laid out like this:
Kids, working alone or in pairs, should draw 18 cards at random from the deck.
Black cards are positive. Red cards are negative
Ace = 1 Jack = 11 Queen = 12 King = 13
Kids need to use these 18 cards to somehow fill in the 14 blanks on their board to make 4 correct equations.
So a completed board would look like
Things to keep in mind:
1) As kids try to fill out their board, they are mentally testing dozens of addition and subtraction problems as they go. Sometimes kids will get three correct equations, only to realize they can't make a correct fourth equation and they have to destroy and rebuild their entire board. If you gave kids this many problems on a worksheet, they would revolt. But they persist because they want to win the game!
2) The colored cards are, in some ways, even more abstract representations of integers than numbers like 4 and -3. Looking at a red queen and thinking -12 is a leap, which is why this is a good end-of-unit activity as opposed to a beginning-of-unit activity. If you wanted to lower the barrier to entry, you could print out slips of paper with integers printed on them.
3) If a kid or a pair of kids completes the activity, tell them "Great work! Ok, now shuffle and deal yourself 17 cards and try again." I have seen kids complete this activity with 16 cards.
I love this game because it doesn't require a lot of supplies, can be played in 15 minutes, and remains challenging even after a student has mastered integer addition and subtraction. Please play with your students and let me know how it goes!
Extra Credit: I picked the starting amount of cards on intuition. I have no idea whether all combinations of 18 cards are solvable in this game. But I have played this game for five years with dozens of students, and I have yet to see a combination of 18 cards that is unsolvable. Even so, I don't know how to prove that 18 cards is always solvable. Any ideas?